Scrolling through my google reader I saw a quick, short post from David Wees asking how do we encourage more questions and the following image:
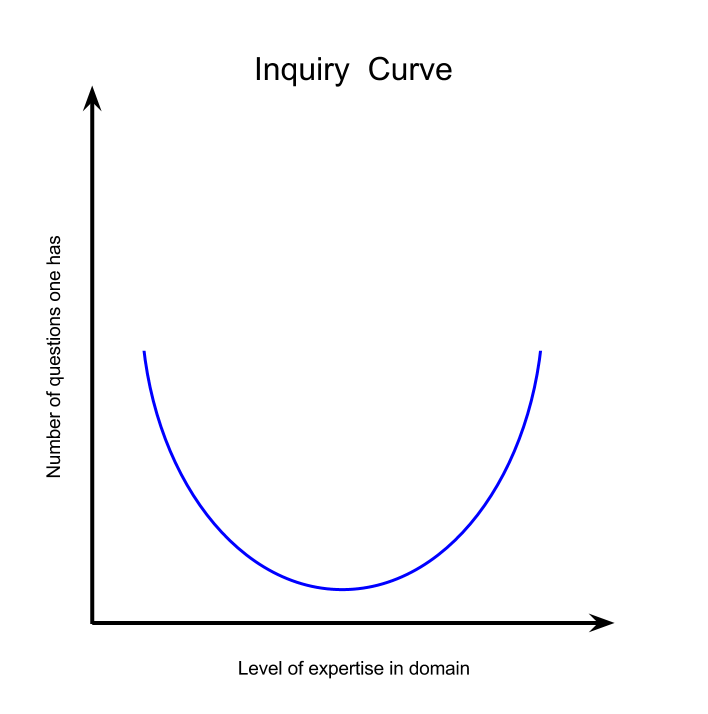
I am a teacher that believes in inquiry learning and also the power of a student driven project or product, but usually these occur as a summary or summative of learning. However, after my recent experiment of completing the Barbie Bungee activity at the start of my unit and in a non-worksheet format, I have seen and appreciate the power of exploring ideas and concepts through the unknown. This activity had my students exploring the ideas of linear equations without knowing what a linear equation was.
They asked brilliant questions including:
- Does the height of the Barbie matter?
- Should I find out how much she weighs?
- Are all the elastics pre-stretched? How can I make sure that they are all pre-stretched the same amount?
- Can I cut my elastics up into smaller parts?
Now, only 9 classes after that activity where students didn’t know what they were looking for, they are now appear to be automatically programed into trying to find a constant rate of change and a starting point (y=mx+b) in each of the problems we look at. As they have gained a greater understanding of their topic they are not asking the insightful questions from before but instead are wondering why I didn’t leave the space on their table of values to include the zero starting value, or if they need to capitalize their variables in an equation. I don’t know if I should be celebrating or concerned.
I also just finished being apart of a webinar by Roger Schank, author of Teaching Minds who shared his view of how he feels that Algebra’s sole purpose is as a method to easily test students, and make benchmarks for university entrance exams. He feels that the multiple steps and multiple areas to have an error do not benefit a student, but only the adults and institutions who require an easy way to grade and place students by how they solve for a variable or express a pattern in the appropriate standard formula.
I know that my students participating in Barbie Bungee activity didn’t need to know the point-slope form or y-intercept form in order create a table, graph the data and find a pattern but they did it anyways. I also know that they used their algebra skills to create a formula or relationship to determine how many elastics they needed to use, all with levels of success. All of this great learning happened, but was followed up with 9 in class days to ensure that they understood what slope meant, how to calculate it in the 3 ways it could be presented in a standard question and how it connects to a graph of a line.
At the end of the day, I am left questioning… do my students know more now that they have been provided the vocabulary and structure to work with linear relations, or have I put a stop on their creative application of the concepts. As a class we will be doing more STEM-like activities and I will be looking to see what my students go to first as their method to solve these new challenges. Will they begin by breaking down the big problem into smaller ones? Will they play with the materials and ask questions to determine if they can find any pattern? Or will they think in what way a linear pattern could be created and what the start and change values should be. Will their questioning level increase or have they reached a level of understanding where they have no desire to explore and think through the problems thoroughly.


Ruth,
I really like this post because it raises great questions about questions. Your last few questions could actually be posed to the students themselves to determine what they are thinking about how the think (metacognition).
The Teaching Center at the Washington University has a great way of setting up questions, and even responses: http://teachingcenter.wustl.edu/asking-questions-improve-learning
Monitoring how students approach problems seems to be your next step, but critically questioning their methods should also be a part of the process.
Thanks for the great post!
garth.
I love the graph. It illustrates nicely the journey that is learning and where inquiry fits in. I found myself reflecting the factors that contribute to the development of expertise and how there exists a point when you can be content but pushed a little further it can open up a whole new world of questions. Some of the questions that you raise at the end of the post might find answers in some sort of exit survey. The challenge there will be in the careful crafting of the questions so they may yield insight into the learning that has occurred.